Find the direction and magnitude of the net electrostatic force

Let q 24 uC and d. No the first one.

Find the magnitude and direction of the net force on a 2-nC charge when placed at the following locations.

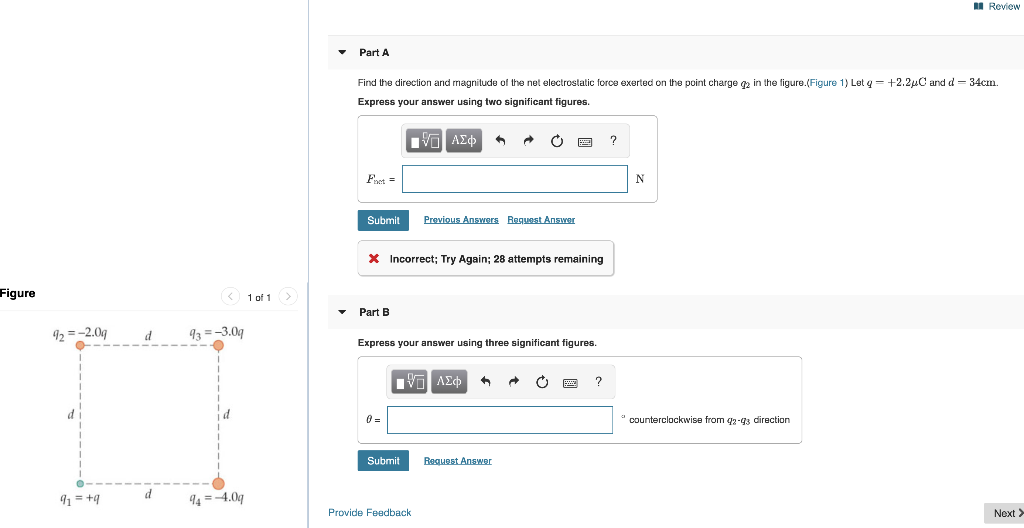
Find the direction and magnitude of the net electrostatic force. Find the direction and magnitude of the net electrostatic force exerted on the point charge q_2 in the figure. Let q 24 uC and d. For Teachers for Schools for Working Scholars.

The magnitude of the net electric force is now found by. Eqbeginalign F_net sqrtF_x2F_y2 sqrt229 N2139 N2 268 N endalign eq The direction. This formula gives the magnitude of the net electrostatic force.

The direction of the net electrostatic force is given by ϴ tan-1 FxFy Here the net electrostatic force on the charges is calculated by adding the individual vector forces that exert the same force on the other charge that is the force exerted by q1 on q2 and force exerted by charge q2 on the q1. Q₁ q 19 µC. Q₂ -20q -38 µC.

Q₃ 30q 57 µC. Use the formula for the electrostatic force. Put in the values for q₁ and q₂ then compute the force on q₁ from q₂.

Do the same with q₁ and q₃. Add the two forces being careful about signs. Each of the two charges on the circle exert a force on the center charge.

Find those forces magnitude and direction using Coulombs law. Perhaps youve done this already Now add them remembering that they are vectors to find the total force. Find the x and y components then use some trig to find the total force and the angle it makes.

Find the direction of the net electrostatic force exerted on the point charge q3 in the figure. Let q 21 μC and d27cm. Find the magnitude of the net electrostatic force exerted on the point charge q3 in the figure.

Let q 21 μC and d27cm. How would your answers to part A and B change if the distance d were doubled. Find the magnitude and direction of the net force on a 2-nC charge when placed at the following locations.

A halfway between the two b half a meter to the left of the charge c half a meter above the charge in a direction perpendicular to the line joining the two fixed charges. A point charge of 30010-6 C is 120cm distant from a second point charge of -15010-6 C. Calculate the magnitude of the electrostatic force on each charge.

Given Q1 30010-6 C Q2 -15010-6 C r 12 cm 012 m. To Find Magnitude of Electrostatic Force F Solution. What are the magnitude and direction of the electrostatic force which acts on the charge at the origin.

Homework Equations F k Q1Q2r2 The Attempt at a Solution F12 90e9 20e-6 20e-6 1m2 036N F13 90e9 2oe-6 20e-6 05m2 144 N Magnitude. When two opposite point charges are placed close to each other the direction of force is positive to negative. The magnitude is merely the value of F without the sign.

According to the above equation F vanishes when r. Hence at an infinitely large distance the electrostatic force is zero. Technically the range of F is infinite.

Rank the arrangements according to the magnitude of the net electrostatic force on the particle with charge Q greatest first. Q going on left of -x axis then charge p at origin then charge p at the top of y axis. Distance of -x axis is 2d and distance of y axis is d.

Provide step by step calculations. Find the direction and magnitude of the net electrostatic force exerted on the a point charge q2 in the attached drawing. Let q 24 micro C and d 33 cm.

B Find the direction and magnitude of the net electrostatic force exerted on the point charge q3. Direction of electric field and electrostatic force should be same by the equation vecF frack q q_0r2 Electric Field vecE frack qr2 Let us suppose that there is a Stack Exchange Network. Once you plug in the right values you should now have a force in the x direction a force in the y direction and a force at an angle of 45º because it was a diagonal of a square.

Using these values calculate the magnitude of Fnet. So F net 115 N. And the angle using SOH CAH TOA.

So θ -3309º. Electrostatic force is proportional to the product of the amount of charge on each interacting object Magnitude of the Electrostatic Force is given by Coulombs Law. F K q 1q 2r2 Coulombs Law where K depends on the system of units K 899x10 9 Nm 2C 2 in MKS system K 14 πε0 where ε0 885x10-12 C 2Nm 2 Electric Charge.

Physics questions and answers. Part A Given that q 12 μC and d 23 cm find the direction and magnitude of the net electrostatic force exerted on the point charge g in the figure. Figure 1 Express your answer using two significant figures.

F 2 k 3 10 9 5 10 9 001 2 898 10 9 3 10 9 5 10 9 001 2. Angle made by the net force with positive x-axis 180 taninverse 13470 10 9 299333 10 9 180 7747 25777 degrees. Magnitude of force 13470 2 299333 2.

A point charge of 1200 x 10-9 C is 15m distant from a second point charge of 680 x 10-9 C. Calculate the magnitude of the electrostatic force on each charge Q1 1200 x 10-9 C Q2 680 x 10-9 C r 15 m F 899 x 10 9 x 1200 x 10-9 x 680 x 10-9 15 2 F 8990000000 x. Find the magnitude of the net electrostatic force using Coulombs Law - YouTube.

And the falls that its asserted by 31. So these are the two forces. So the magnitude of the force or the net force will be the some of this too.

No the first one. First were going to calculate the magnitude. So the magnitude of the force 21 is columns constant times um the charge Q one times Q two over the distance as square.

For case of more than two forces the net force vector is FFFF net 12 3. JG JG JG JG The X and Y components of the net force are FFFF netxxx x1 2 3. FFFF net y y y y1 2 3.

The magnitude and direction of the net force are 22 FF F net net x net y 1 θ tan FF net y net x. Answer to a Find the direction and magnitude of the net electrostatic force exerted on the point charge q3 in Figure 19- 41. Let q 24 µC and d 27 cm.

B How w SolutionInn. The individual forces magnitude should be known before calculating the net force acting on a body. The force is calculated by using the formula derived from Newtons Second Law of Motion.

Here m mass of the body. A acceleration produced by force.